Journal of Chemical Sciences | Lupine Publishers
Abstract
Introduction
Other options for the shape of the occupancy numbers result from the different associated functional with finite temperature to DFT but without physical meaning, such as the temperature or the entropy associated with this term [3]. These terms, although numerically small must be included in the practical calculations that allow numbers of fractional occupation [3,8]. To consider the scope of smearing, it is known that electrons occupy orbitals with the lowest energies, and occupancy numbers are integers; nonetheless, there is a need for a fractional occupation in virtual orbitals within this space of occupation. We apply this when the HOMO-LUMO gap is small and there is especially a significant density near of Fermi level [9], thus in order to obtain the fractional occupation a kT term is implemented. This fractional occupation pattern depends on the temperature. The systems C48 carbinoid, C24 carbyne-ring, and C9 cumulene-ring (almost-planar) are arrangements obtained through DFT geometry optimization of two hypothetical parallel zigzag linear carbon chains. We consider these systems as carbon physically activated, due to the pore size diameter, and since no activating chemical agent has been applied. Carbyne is known as linear carbons alternating single and triple bonds (-C≡C-) n or with double bonds (=C=C=)n (cumulene) [10]. Polyyne is known as a allotrope carbon having H(-C≡C-) nH chemical structure repeating chain, with alternating single and triple bonds [11] and hydrogen at every extremity, corresponding to hydrogenated linear carbon chain as any member of the polyyne family HC2nH [12] with sp hybridization atoms. It is known that polyyne, carbyne and carbinoid have been actually synthesized as documented by Cataldo [13]. Bond length alternation (BLA) of carbyne pattern is retained in the rings having an even number of atoms [10]. Additional care must be taken with carbyne rings since the Jahn-Teller distortion (the counterpart of Peierls instability in non-linear molecules) is different in the C4N and C4N+2 families of rings [14-16]. There is a great variety of applications of activated carbon as an adsorbent material, and it has been used in areas related to the energy, and the environment, generating materials with a high-energy storage capacity [17].
Chitin is, after cellulose, the most abundant biopolymer in nature. When the degree of deacetylation of chitin reaches about 50% (depending on the origin of the polymer), it becomes soluble in aqueous acidic media and is called chitosan [18]. Chitosan is applied to remediation of heavy metals in drinking water and other contaminants by adsorption. The affinity of chitosan with heavy metals makes the bisorption process stable and advantageous, being only by the alginates present in brown algae matched [19]. The glass transition temperature of chitosan is 203°C (476.15 K) according to Sakurai et al. [20], 225°C (498.15 K) according to Kadokawa [21], and 280°C (553.15 K) according to Cardona-Trujillo [22]. One can differentiate specific reactions involving the -NH2 group at nonspecific reactions of -OH groups. This is important to difference between chitosan and cellulose, where three -OH groups of nearly equal reactivity are available [23,24]. In industrial applications, several solids having pores close to molecular dimensions (micropores < 20 Å) are used as selective adsorbents because of the physicochemical specificity they display towards certain molecules in contrast to the mesoporous substrates (20-500 Å) and macropores (> 500 Å). Adsorbents with these selective properties include activated carbon among others [25]. Chitosan-based highly activated carbons have also application for hydrogen storage [26]. In principle, electronic structure of diatomic molecules has been built through the overlapping knowledge of the interacting atomic orbitals [27]. In this case, the orbitals correspond to bonding (σg, πg) and antibonding (σu, πu) orbitals of hydrogen, carbon, nitrogen and oxygen diatomic molecules, whose H2, C2, N2, and O2 groundstate electronic configurations are
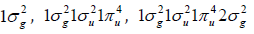and 
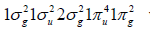with 2, 8, 10 and 12 valence electrons,
respectively. Actually, the reactivity sites in a molecule correspond
to the highest occupied molecular orbitals (HOMO) and lowest
unoccupied molecular orbitals (LUMO). HOMO as base (donor), and
LUMO as acid (acceptor) are particularly important MOs to predict
reactivity in many types of reaction [28,29]. Activated carbon and
chitosan have been independently applied as sorption materials to
increase environmental quality standards. Then, we expect AC-Ch
nanocomposite to have a powerful handleable adsorption property
of pollutants that can be applied not only in wastewater treatment,
but also in medicine against intoxication, in batteries to increase
storage capacity, in electrodes of fuel cells, and in more possible
applications, according to the pore size distribution to be generated
on this new material.Methodology
Single point potential energy curves were constructed [1,2] by using smearing. The following conditions to find AC+Ch (Activated Carbon+Chitosan) interaction energy are: functional GGA-PW91 [31,33-36], unrestricted spin, dnd bases, and orbital occupation with various smearing values. Considering that we obtained a solution for the energy value convergence, the interaction by changing the smearing value was studied. Since electrons occupy orbitals with lower energies and integral occupation numbers in calculations of density functional, a smearing change indicates fractional occupation and virtual orbital within this occupation space [19]. When generating a fractional occupation, virtual orbitals are in this occupation space generated, if the HOMO-LUMO gap is small, and there is certain density near the Fermi level [1], then it is implemented the fractional occupation term kT. This pattern of fractional occupation depends on temperature. Covalent connectivity calculations [37] according to DMol3 on no-bonding to s- and f-shell scheme, bond type, and converting representation to Kekulé, for bond length tolerances from 0.6 to 1.15 Ǻ were accomplished in this molecular complex mostly composed of carbon. Area calculations have been carried out by inserting triangles in each amorphous carbon ring and using the
Heron formula:
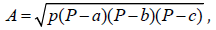where P=(a+b+c)/2
is the perimeter of a triangle of a, b, c sides; while the pore size
diameter (PSD) is calculated as an approximation to the circle area.
Periodic systems can be constructed using amorphous builder
of BIOVIA Materials Studio, these are useful to calculate Radial
Distribution Functions and the area under the curve on a significant
interval.Results
Chitosan Optimized by Applying Smearing
The default smearing value of 0.005Ha corresponds to T=1578.87 K and P=224.806 atm. We now exhibit electron smearing behavior using the known Fermi-Dirac statistic [38]. Facing two hydrogen atoms and using geometry optimization calculations, we built energy as a function of smearing value. Figure 1 shows the total energy variation when the system is optimized with respect to smearing value [39] (Figure 1). The fractional occupational pattern depends on the temperature, and this is derived from the energy change of Fermi distribution [6] as: 𝛿𝐸 = 𝑇𝑘; where k is Boltzmann constant. Considering a model in which the electrons are free and given that clouds of electrons are being a Fermi gas considered. The pressure is: 2/3 δE/δV [38]. From the latter two previous equations, temperature and pressure change is observed in Table 1 given the 𝛿𝐸 smearing energy. The planar molecular hypothetical system of 48 carbons is built by applying geometry optimization at two linear chains of 24 carbons as shown in Figure 2a, and the chitosan copolymer molecular system is built without applying geometry optimization, as observed in Figure 2b. Approaching enough these two molecular systems we studied a new molecular complex at different smearing values. The molecular model of carbon is symmetrically arranged in planar geometry, and it is physically activated through geometry optimization. We called activated carbon (AC) to the resulting planar carbon system. The length of this planar system is comparable to that one of chitosan (Ch). Each six-carbon ring has an area 4.34 Å2, each eight-carbon ring along with this has an area 8.74 Å2, each eight-carbon ring along with the sixteen-carbon ring has an area 8.55 Å2, and the sixteencarbon ring has an area 27.32 Å2. Considering each one of this area as circle areas the pore size diameter distribution is from 2.35 Å to 5.9 Å, which correspond to micropore size distribution of this carbon system. When considering the whole area of this system for calculating the pore size diameter 9.48 Å [40,41]. Chitosan is very well known to be macropore size [42] (Figure 2
Figure 1: Change in the total energy as a function of the electron smearing value [39].
Figure 2: C48 Carbon and Chitosan molecular systems. a) Input-Output of a C48 carbon system geometry optimization. Carbon
atoms in gray color. b) Chitosan molecule (C14H24N2O9) without optimization. Hydrogen atoms in white color, Nitrogen in blue
color and oxygen in red color.
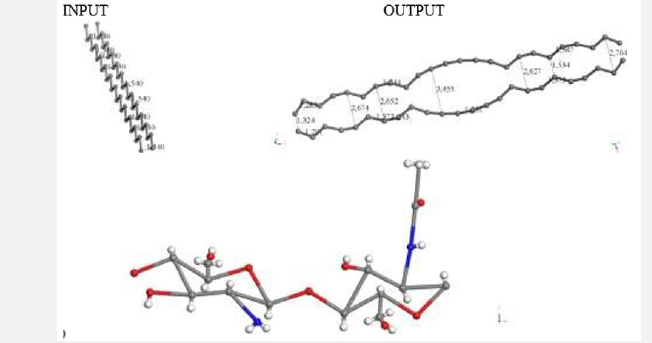
Figure 3: INPUT for interaction among activated carbon (AC) and one copolymer unit of Chitosan (Ch). a) Chitosan without
geometry optimization. b) Potential energy curve with well depth of 30Kcal/mol for smearing: 0.05Ha. c) Potential energy
curve with well depth of -1089 kcal/mol for smearing of 0.03 Ha.
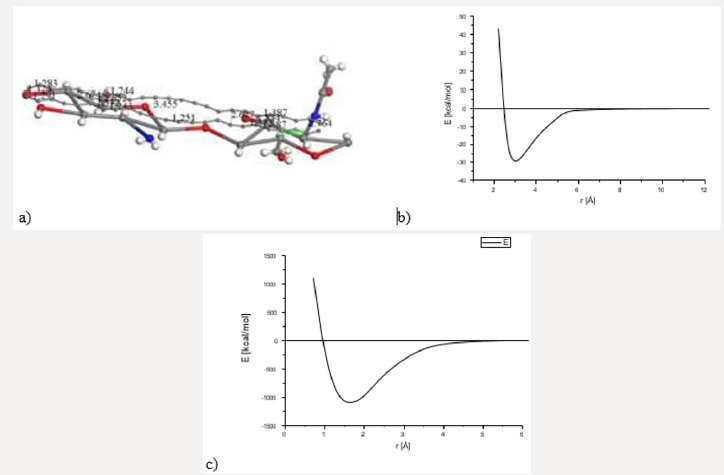
Searching for a new molecular complex, Figure 3 exhibits the
potential energy curve of the interaction between AC and Ch having
equilibrium at (1.6Å, -1089Kcal/mol). In this case chitosan was not
geometrically optimized in order to build the potential energy curve
observed in Figures 3b & 3c. It was really easy to build this curve
using smearing energy 0.05 Ha for every single point calculated, and
hard to build it at 0.03 Ha. We also tried lower values than this,
and we obtained poor or none results (Figure 3). After applying
geometry optimization at smearing 0.05 Ha, and subsequently at
0.03 Ha. The smearing at 0.02 Ha is shown in Figure 4a. Then, we
built the potential energy curve as shown in Figure 4b in step by
step single point calculations for AC + Ch face to face interaction,
when 2.264 Å is the separation between their corresponding
centers of mass. The latter has a potential well depth of 165 Kcal/
mol at a distance of 2.2 Å, meaning formation of a new molecular
complex at an adsorption energy greater than 20 kcal/mol in the
chemisorption range [43] (Figure 4). Covalent connectivity [37] to
the resulting system in Figure 4a was applied under the conditions
previously mentioned in methodology, and the molecular complex
observed in Figure 5 is obtained. In this complex the reactants and
products are C48 + C14H24N2O9 and C49H3O3 + CH2 + C4H6O2 + CH3NO
+ C2H2O + CH2O + C2H2 + CHNO + CH3, respectively. Carbon bonds
are single, double, and triple, as an example the C12 ring has eight
double bonds, one triple bond, and three single bonds, where all
the carbon valence electrons are shared. Furthermore, C8 and C16
rings have double bonds in one side of the ring, and single and
triple bonds in the other side; and C6 ring has four double bonds
and two single bonds. This whole carbon system has been activated
by chitosan, and double bonds, and single and triple bonds are the
representative characteristics of carbine-type molecules (Figure 5).
Figure 4: OUTPUT for interaction among activated carbon (AC)and one copolymer unit of Chitosan (Ch) after DFT geometry
optimization using smearing at 0.02Ha.
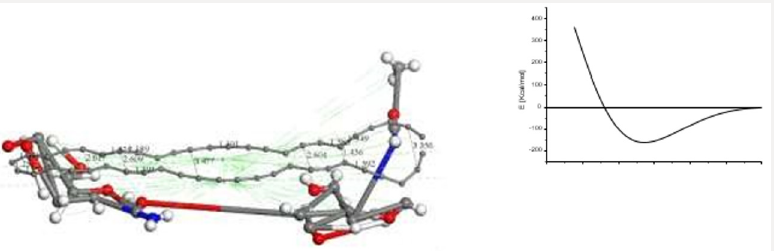
Figure 5: Connectivity applied after geometry optimization of CA+ Ch interaction (smearing at 0.02 Ha)
It must be noticed that geometry optimization of this whole
system provides a lowest unoccupied molecular orbital (LUMO
- electron acceptor) receiving an electron pair from the highest
occupied molecular orbital (HOMO - electron donor). The donor
HOMO from the base and the acceptor LUMO from the acid, combine
with a molecular orbital bonding, which in our case corresponds to the orbitals 242-HOMO for E=-0.18317 Ha and 243-LUMO for E=-
0.17786, for a Fermi energy of -3136.28 Ha with A as irreducible
representation of symmetry C1. The total orbitals number is 274.
The orbital occupation is 202 A (2) plus 78 electrons in 65 orbitals,
for a total number of 482 active electrons and binding energy of
-22.997 Ha, at 2 steps. However, in order to get HOMO and LUMO
drawn in this model, we run an energy calculation. Then, this
molecular complex as seen in Figures 6a & 6b has HOMO-484 with
E=-0.16398 Ha, LUMO-485 E=-0.16196 Ha, and Fermi energy Ef =
-3161.44 Ha, for the reactivity sites with 482 active electrons. The
total number of valence orbitals is 1070. The orbital occupation is
206 A (1) alpha and 206 A (1) beta, and 35.00 alpha electrons in 62
orbitals plus 35 beta electrons in 62 orbitals. HOMO as base-donor,
and LUMO as acid-acceptor are the MOs locating possible reactivity
in this reaction. An acid-acceptor can receive an electron pair in its
lowest unoccupied molecular orbital from the base-donor highest
occupied molecular orbital. That is to say, the HOMO from the base
and the LUMO from the acid combine with a bonding molecular
orbital in the ground state see Figure 6c.
Figure 6: We applied highest occupied molecular orbital (HOMO) and lowest unoccupied molecular orbital (LUMO) to the
previous AC+Ch molecular complex. a) HOMO. b) LUMO. c) HOMO-LUMO. Blue and yellow isosurfaces of the HOMO and
LUMO denote positive and negative wave function phases, respectively.
Figure 7: After covalent connectivity and another geometry optimization at smearing 0.02 Ha we mostly obtain highest
occupied molecular orbitals a) HOMO; and we scarcely obtain lowest unoccupied molecular orbitals b) LUMO. The most
molecular orbitals c) HOMOLUMO correspond to bonds of carbon atoms.
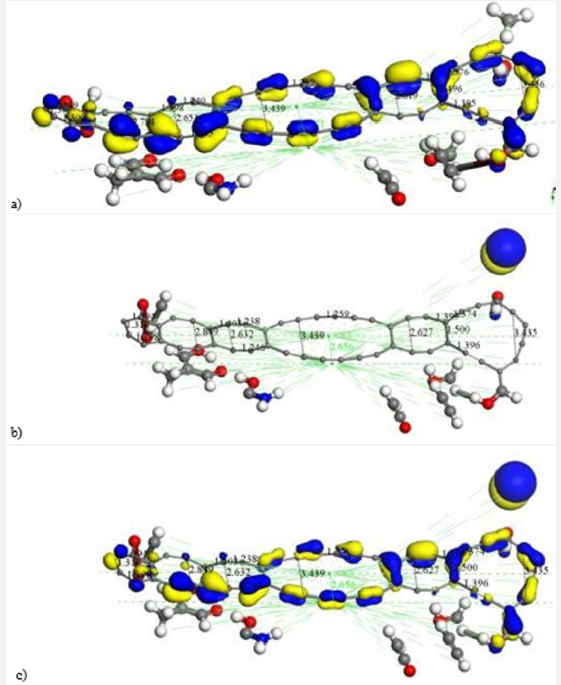
After applying covalent connectivity [37] to the resulting system
in Figure 6, we again applied geometry optimization for smearing
0.02Ha, and we obtain different molecular orbitals in the results,
as shown in Figure 7. This molecular complex as seen in Figure 7
has HOMO-482 with E=-0.17650 Ha, LUMO-483 E=0.16060 Ha, and
Fermi energy Ef = -3162.004 Ha, for the reactivity sites with 482
active electrons. The orbital occupation is 204 A (1) alpha and 204
A (1) beta, and 37.00 alpha electrons in 62 orbitals plus 37 beta
electrons in 62 orbitals. The molecular complex observed in Figure 7
has the same products previously mentioned. It must be noticed that
the lowest unoccupied molecular orbitals (LUMO-acceptor) only
draw orbitals in the CH3 product, the rest of the molecular orbitals
correspond to the highest occupied molecular orbitals (HOMOdonor)
complex. Then, this is a very stable molecular system only
allowing reactivity through the methyl radical CH3 (Figure 7) The
potential energy curve in Figure 3b is very near to physisorption;
however, smearing energy in this case corresponds with a very high
temperature, which actually occurrs little inside sun surface. In
this work, we gradually get down smearing energy searching until
reaching the glass transition temperature of chitosan. The smearing
energy value 0.02 Ha corresponds with temperature 6315.49 K
according to Table 1, and it is still too high; however, is this way we
have been achieving geometry optimization to reach right smearing
values according to experimental measurements. After successful
convergence in geometry optimizations at 0.01, 0.007, 0.005,
0.003, and 0.002 smearing energies, the convergence at smearing energy 0.0017 Ha has been unsuccessful after more than 10000
SCF iterations for an oscillating energy with an energy tolerance
of 0.00002 Ha. After these calculations, we continued rising the
smearing energy until 0.00175, and after more than 5000 SCF,
convergence is successfully accomplished. The temperature 552.6
K reached for smearing at 0.00175 agrees with glass transition
temperature range [498.15K, 553.15K] of chitosan, according to
experimental measurements [20-22].
Table 1: Change of temperature and pressure due to smearing variation 𝛿𝐸[𝐻𝑎] at temperature T [K] and pressure P [atm], for a
volumen of 638 Å3.
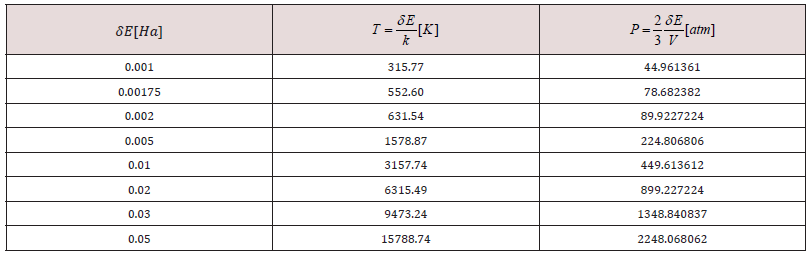
Figure 8 illustrates the final stage of the molecular complex
formed. We can observe that while C48 has been deformed mainly in
its planarity, the chitosan ended broken in the two initial groups of
each polymer, also apparently divided in several smaller molecules.
This fact is very well known experimentally, because one bonding
solution (epichlorhydrine, glutaraldehyde, or EGDE -ethylene glycol
glycidyl ether-) is commonly used to keep chitosan copolymer
cross-linked for enhancing the resistance of sorbent beads against
acid, alkali, or chemicals [19]. The products observed by applying
covalent connectivity (under the bonding scheme for no bonding to
s- and f- shell, covalent connectivity and bond type, and converting
representation to Kekulé) are the following: C51H7NO4 + C4H6O2 +
C2H2O + C2H2 + CH3O + CHNO + CH3. As it can be seen part of each
polymer remain bonded to the AC system (Figure 8). Then, at
smearing 0.00175 Ha we mostly obtain highest occupied molecular
orbitals for the molecular complex observed in Figure 9. This output
exhibits the orbitals a) HOMO-482 with an eigenvalue of -0.17013
Ha, b) LUMO-483 with an eigenvalue of -0.16923 Ha, and c) HOMOLUMO.
The Fermi energy is Ef = 3162.0047053 Ha, for the reactivity
sites with 482 active electrons. The orbital occupation is 238 A (1)
alpha and 239 A (1) beta, and 2.96 alpha electrons in 5 orbitals plus
2.04 beta electrons in 4 orbitals (Figure 9)
Figure 8: OUTPUT of the AC+Ch interaction after geometry optimization using smearing at 0.00175 Ha, corresponding to
552.6 K and 78.68 atm according to Table 1.
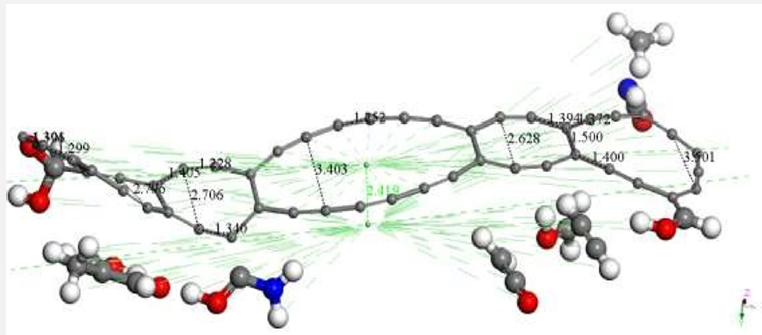
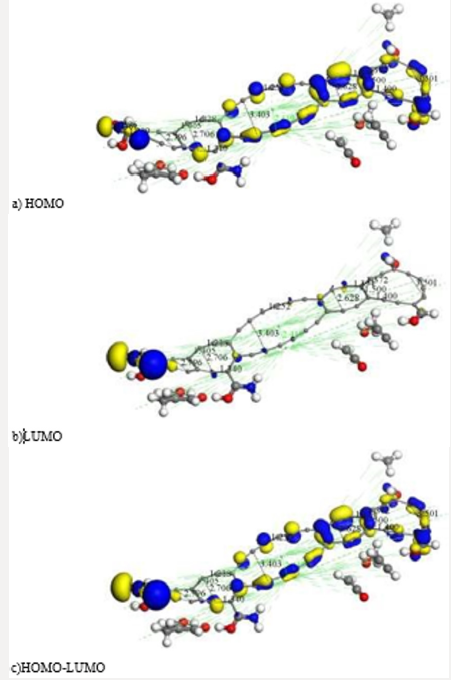
Figure 9: After another geometry optimization at smearing 0.00175 Ha: a) we mostly obtain highest occupied molecular orbitals
HOMO, b) we scarcely obtain lowest unoccupied molecular orbitals LUMO, c) the greatest part of molecular orbitals HOMOLUMO
correspond to bonds of carbon atoms.
Chitosan Optimized Without Smearing
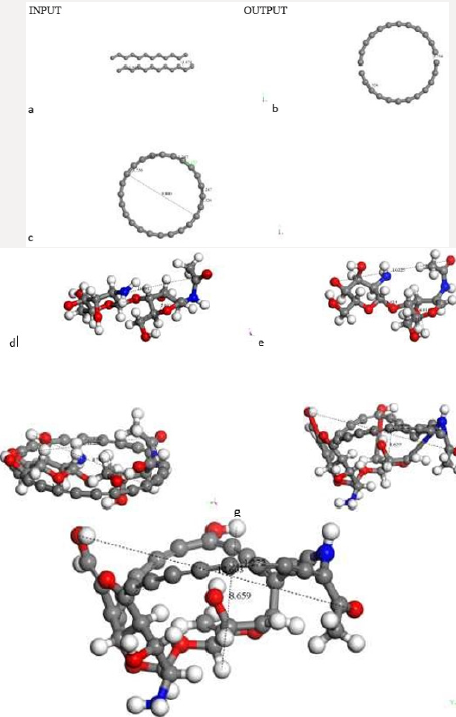
First of all, the C24 carbyne-type ring alternating single and triple bonds is obtained by applying connectivity [37] and bond type to a C24 carbon ring which is the output of the input shown in Figure 10a corresponding to the geometry optimization of two hypothetical C12-carbon chains (Figure 10b). Then, Figure 10c exhibits an alternating single and triple bonds C24-ring. Second, applying clean of BIOVIA Materials Studio on chitosan copolymer molecule designed in Figure 2b, we obtain the input of a chitosan copolymer molecule as in Figure10d, and the Output exhibiting geometry optimization of the previous molecule is shown in Figure 10e. As we can observe, in this case chitosan remained complete. We made this, after suspecting that the initial bonds lengths and angles were not right in our design of chitosan, because broken chitosan is not a satisfactory result. Then, mixing the optimized C24 and Ch systems as shown in Figure 10f in the Input of a C24-ring surrounding a chitosan copolymer molecule, and after applying geometry optimization we obtain the Output of the previous CA-Ch nanocomposite see Figure 10g. Finally, we applied bonding scheme criteria as in Figure 10h.The nanocomposite in Figure 10h is a good example of the possibility of modifying the pore size distribution of chitosan when it is embedded into activated carbon. Here we consider INPUT and OUTPUT for applying geometry optimization on activated carbon and chitosan C14H24N2O9 system after each part has been previously optimized, and we also applied bond criteria for connectivity, bond type and kekulé representation. The C24-ring is carbyne type, and the chitosan copolymer molecule has been optimized in three dimensions in this case. The position of C24- ring surrounding a chitosan copolymer molecule has been only proposed.
Figure 10: Here we consider INPUT and OUTPUT of the corresponding geometry optimization, and also applying bond criteria
for connectivity, bond type and Kekulé representation. a) Input among two hypothetical C12-carbon chains. b) Output showing
a disconnected C24-ring. c) The previous C24-ring linked using bond criteria. d) Input of a chitosan copolymer molecule. e)
Output exhibiting the optimization of the previous molecule. f) Input of a C24-ring surrounding a chitosan copolymer molecule,
g) Output of the previous CA-Chitosan, h) Bonding criteria applied to the previous output
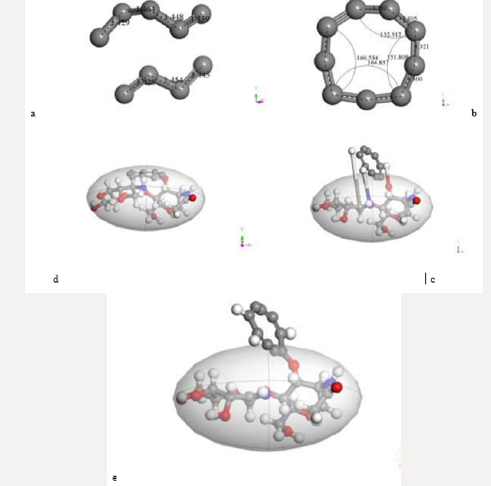
From the interaction through geometry optimization of two
linear carbon chains of four and five carbon atoms as in Figure 11a,
cumulene C9-ring shown in Figure 11b is obtained. This is a clear
evidence of Jahn-Teller effect, because we observe double bond
lengths alternating long/short with a difference among .02 and .03
Å, and the angles in this non-planar (Figure 11b) cumulene molecule
are also different. The expected angles in a planar symmetrical
molecule should be the same according to a well-defined symmetry.
We considered the interaction of chitosan with another almost
planar carbon ring of nine carbon atoms, now one in front to the
other as in Figure 11c. Then, in Figure 11d there is another example
about building pore size distribution among chitosan and activated
carbon. In this case, we consider INPUT and OUTPUT for geometry
optimization of cumulene C9-ring and chitosan C14H24N2O9, each one previously optimized by applying geometry optimization
to the whole system, and also considering the bond criteria for
connectivity, bond type and Kekulé representation as shown in
Figure 11e. The cumulene C9-ring and chitosan copolymer molecule
have been optimized in three dimensions, and we clearly observe
the cumulene passing from face to face to almost T-shape orientation
taking three hydrogen atoms from chitosan. The input position of
cumulene C9 ring face to face with chitosan in that precise location
has been proposed, and the result has been excellent.
Figure 11: Here we consider INPUT and OUTPUT of the geometry optimization among a cumulene C9-ring and a Chitosan
C14H24N2O9 molecule, and also applying bond criteria for connectivity, bond type and Kekulé representation. a) Input among
a hypothetical C4- and C5- chains. b) Output showing a C9-ring. c) Input among the C9-ring and chitosan molecule. d) Output
exhibiting the complex C9-ring into chitosan. e) Bonding criteria applied to the previous output.
Discussion
The strongly dependence on smearing means very closely spaced energy levels (high degeneracy) near Fermi level. When there is a degenerate electron state, any symmetrical position of the nuclei (except when they are collinear) is unstable. As a result of this instability, the nuclei move in such a way that the symmetry of their configuration is destroyed, the degeneracy of the term is being completely removed [44,45]. High degeneracy indicates a high symmetry of the molecule, then the system tends to be distorted, in such way that when moving, the occupied levels are down and the unoccupied ones are up [46]. When levels are very densely spaced, convergence is hard to reach, since very small changes will occupy completely different states, and we get oscillations. These can be damped by smearing out the occupancy over more states, so that we turn off the binary occupancy of the states. We get down smearing width to glass transition temperature by decreasing the smearing parameter in steps to gradually stabilize our molecular complex system at the right temperature.
We initially observe distortion of chitosan system, and then its possible breaking in some products. This is partially in agreement with the results presented by Chigo et al. [46] in a study of the interaction among graphene-chitosan for a relaxed system doped with boron, in which they consider the interaction of pristine graphene with the monomer of chitosan (G + MCh:C6H13O5N) in different configurations, whereas we consider a chitosan copolymer molecule: C14H24N2O9 in only one orientation. While Chigo et al. [46] found a perpendicular chitosan, molecule linked to a carbon nanotube system, we obtained a cumulene carbon ring almost perpendicularly linked to a chitosan copolymer molecule.
Conclusion
For more Lupine Publishers Open Access Journals Please visit our
website:https://lupinepublishersgroup.com/
For more Journal of Chemical Sciences Please Click Here:
To Know More About Open Access Publishers Please Click on Lupine Publishers
No comments:
Post a Comment
Note: only a member of this blog may post a comment.